Morphology of flowering plant mind map
Welcome to my Rushikesh Vishnu Adalinge blogger I will give you all 10th and 11th std mind map or question answer please support me you have any problem please tell me in the comment box. Gmail:-rushikeshadalinge70@gmail.com Telegram channel :-@Rushikesh123455
Sunday, November 28, 2021
Wednesday, November 24, 2021
Structure of atom mind map
Structure of atom mind map for class 11th also use in neet/jee exam
*Quantum Number*
1)Principal quantum no(n)
this quantum number determines the main energy level or sell to which electron belongs and have values of n n=1,2,3,4
n value can never be zero
2n^2
n=1. 2e
n=2. 8e
n=3. 18e
n=4. 32e
2) Azimeuthal quantum number(l)
It is it is also called secondary angular or quantum no.number it gives the subshell to which electron belongs
Allowted values 0 to n-1
If n=1. The values l=0
n=2. The values l=0
1
n=3. The values l=0
1
2
n=4. The values.l=0
1
2
3
When l=o then it is the *s* subshell
When l=1 then it is the *p* subshell
When l=2 then it is the *d* subshell
When l=3 then it is the *f* subshell
In every sabse electronica field on the basis of formula =2(2l+1)
Subshell
l=o. 2e. S
l=1. 6e. P
l=2. 10e. D
l=3. 14e. f
3) magnetic quantum number(m)
It is explain zeeman effect
Describe the behaviour of an electron in magnetic
Values of m are (-l through o+l)
Suppose n=3. L. Subshell
O. [] s
1 -1,o,1. [][][] p
2 -2,-1,o,1,2. [][][][][] d
4) spin quantum number(s)
An electron can spin clockwise or anticlockwise that is into two opposite direction
+1/2 and -1/2 orientation are clockwise or anticlockwise
Shapes of the orbital
* s* orbit shaped
Properties
1) d orbital are double dumbbell in shape
2)They are denoted as( dxy dyz dxz dx^2y^2 dz^2)
Tuesday, November 23, 2021
Physics class 11th units and its measurement question and answer
Maharashtra Board Class 11 Physics Solutions Chapter 1 Units and Measurements
Chapter 1 Units and Measurements Textbook Exercise Questions and Answers.
Maharashtra State Board 11th Physics Solutions Chapter 1 Units and Measurements
1. Choose the correct option.
Question 1.
[L1M1T-2] is the dimensional formula for
(A) Velocity
(B) Acceleration
(C) Force
(D) Work
Answer:
(C) Force
Question 2.
The error in the measurement of the sides of a rectangle is 1%. The error in the measurement of its area is
(A) 1%
(B)
(C) 2%
(D) None of the above.
Answer:
(C) 2
Question 3.
Light year is a unit of
(A) Time
(B) Mass
(C) Distance
(D) Luminosity
Answer:
(C) Distance
Question 4.
Dimensions of kinetic energy are the same as that of
(A) Force
(B) Acceleration
(C) Work
(D) Pressure
Answer:
(C) Work
Question 5.
Which of the following is not a fundamental unit?
(A) cm
(B) kg
(C) centigrade
(D) volt
Answer:
(D) volt
2. Answer the following questions.
Question 1.
Star A is farther than star B. Which star will have a large parallax angle?
Answer:
i). ‘b’ is constant for the two stars
∴ θ ∝
ii) As star A is farther i.e., DA > DB
⇒ θA < θB
Hence, star B will have larger parallax angle than star A.
Question 2.
What are the dimensions of the quantity l =
Answer:
l=lxg
[l1T-1] hence proved by p. O. H
[Note: When power of symbol expressing fundamental quantity appearing in the dimensional formula is not given, ills taken as 1.
Question 3.
Define absolute error, mean absolute error, relative error and percentage
appearing in the dimensional formula is not given, ills taken as 1.]
![]()
Question 3.
Define absolute error, mean absolute error, relative error and percentage error.
Answer:
Absolute error:
a. For a given set of measurements of a quantity, the magnitude of the difference between mean value (Most probable value) and each individual value is called absolute error (∆a) in the measurement of that quantity.
b. absolute error = |mean value – measured value|
∆a1 = |amean – a1|
Similarly, ∆a2 = |amean – a2|
. . . ..
. . . .
. . . .
∆an = |amean – an|
Mean absolute error:
For a given set of measurements of a same quantity the arithmetic mean of all the absolute errors is called mean absolute error in the measurement of that physical quantity.
∆amean =
Relative error:
The ratio of the mean absolute error in the measurement of a physical quantity to its arithmetic mean value is called relative error.
Relative error =
Percentage error:
The relative error represented by percentage (i.e., multiplied by 100) is called the percentage error.
Percentage error =
[Note: Considering conceptual conventions question is modified to define percentage error and not mean percentage error.]
Question 4.
Describe what is meant by significant figures and order of magnitude.
Answer:
Significant figures:
- Significant figures in the measured value of a physical quantity is the sum of reliable digits and the first uncertain digit.
OR
The number of digits in a measurement about which we are certain, plus one additional digit, the first one about which we are not certain is known as significant figures or significant digits. - Larger the number of significant figures obtained in a measurement, greater is the accuracy of the measurement. The reverse is also true.
- If one uses the instrument of smaller least count, the number of significant digits increases.
Rules for determining significant figures:
- All the non-zero digits are significant, for example if the volume of an object is 178.43 cm3, there are five significant digits which are 1,7,8,4 and 3.
- All the zeros between two nonzero digits are significant, eg., m = 165.02 g has 5 significant digits.
- If the number is less than 1, the zero/zeroes on the right of the decimal point and to the left of the first nonzero digit are not significant e.g. in 0.001405, significant. Thus the above number has four significant digits.
- The zeroes on the right hand side of the last nonzero
- The zeroes on the right hand side of the last nonzero number are significant (but for this, the number must be written with a decimal point), e.g. 1.500 or 0.01500 both have 4 significant figures each.
On the contrary, if a measurement yields length L given as L = 125 m = 12500 cm = 125000 mm, it has only three significant digits. - Speed of light in air = 3 × 108 m/s
∴ order of magnitude = 8 - Mass of an electron = 9.1 × 10-31 kg
= 0.91 × 1030 kg
∴ order of magnitude = -30
Order of magnitude:
The magnitude of any physical quantity can be expressed as A × 10n where ‘A’ is a number such that 0.5 ≤ A < 5 then, ‘n’ is an integer called the order of magnitude.
Examples:
![]()
Question 5.
If the measured values of two quantities are A ± ∆A and B ± ∆B, ∆A and ∆B being the mean absolute errors. What is the maximum possible error in A ± B? Show that if Z =
Answer:
Maximum possible error in (A ± B) is (∆A + ∆B).
Errors in divisions:
i) A
Suppose, Z = 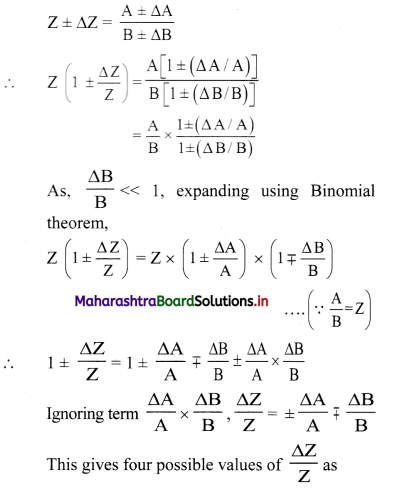
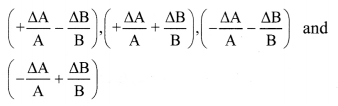
∴ Maximum relative error of
ii) Thus, when two quantities are divided, the maximum relative error in the result is the sum of relative errors in each quantity.
Question 6.
Derive the formula for kinetic energy of a particle having mass m and velocity v using dimensional analysis
Answer:
Kinetic energy of a body depends upon mass (m) and velocity (v) of the body.
Let K.E. ∝ mx vy
∴ K.E. = kmx vy …….. (1)
where,
k = dimensionless constant of proportionality. Taking dimensions on both sides of equation (1),
[L2M1T-2] – [L0M1T0]x [L1M0T-1]y
= [L0MxT0] [LyM0T-y]
= [L0+yMx+0T0-y]
[L2M1T2] = [LyMxT-y] …………. (2)
Equating dimensions of L, M, T on both sides of equation (2),
x = 1 and y = 2 ,
Substituting x, y in equation (1), we have
K.E. = kmv2
3. Solve numerical examples.
Question 1.
The masses of two bodies are measured to be 15.7 ± 0.2 kg and 27.3 ± 0.3 kg. What is the total mass of the two and the error in it?
Answer:
Given: A ± ∆A = 15.7 ± 0.2kg and
B ± ∆B = 27.3 ± 0.3 kg.
To find: Total mass
5
Balbharathi Solutions
Skip to content
Menu
Maharashtra Board Solutions
Menu
Maharashtra Board Class 11 Physics Solutions Chapter 1 Units and Measurements
September 18, 2021 by Prasanna
Balbharti Maharashtra State Board 11th Physics Textbook Solutions Chapter 1 Units and Measurements Textbook Exercise Questions and Answers.
Maharashtra State Board 11th Physics Solutions Chapter 1 Units and Measurements
1. Choose the correct option.
Question 1.
[L1M1T-2] is the dimensional formula for
(A) Velocity
(B) Acceleration
(C) Force
(D) Work
Answer:
(C) Force
Question 2.
The error in the measurement of the sides of a rectangle is 1%. The error in the measurement of its area is
(A) 1%
(B) 12%
(C) 2%
(D) None of the above.
Answer:
(C) 2%
Maharashtra Board Class 11 Physics Solutions Chapter 1 Units and Measurements
Question 3.
Light year is a unit of
(A) Time
(B) Mass
(C) Distance
(D) Luminosity
Answer:
(C) Distance
Question 4.
Dimensions of kinetic energy are the same as that of
(A) Force
(B) Acceleration
(C) Work
(D) Pressure
Answer:
(C) Work
Question 5.
Which of the following is not a fundamental unit?
(A) cm
(B) kg
(C) centigrade
(D) volt
Answer:
(D) volt
2. Answer the following questions.
Question 1.
Star A is farther than star B. Which star will have a large parallax angle?
Answer:
Maharashtra Board Class 11 Physics Solutions Chapter 1 Units and Measurements 1
i). ‘b’ is constant for the two stars
∴ θ ∝ 1D
ii) As star A is farther i.e., DA > DB
⇒ θA < θB
Hence, star B will have larger parallax angle than star A.
Question 2.
What are the dimensions of the quantity l l/g−−−√, l being the length and g the acceleration due to gravity?
Answer:
Maharashtra Board Class 11 Physics Solutions Chapter 1 Units and Measurements 2
[Note: When power of symbol expressing fundamental quantity appearing in the dimensional formula is not given, ills taken as 1.]
Maharashtra Board Class 11 Physics Solutions Chapter 1 Units and Measurements
Question 3.
Define absolute error, mean absolute error, relative error and percentage error.
Answer:
Absolute error:
a. For a given set of measurements of a quantity, the magnitude of the difference between mean value (Most probable value) and each individual value is called absolute error (∆a) in the measurement of that quantity.
b. absolute error = |mean value – measured value|
∆a1 = |amean – a1|
Similarly, ∆a2 = |amean – a2|
. . . ..
. . . .
. . . .
∆an = |amean – an|
Mean absolute error:
For a given set of measurements of a same quantity the arithmetic mean of all the absolute errors is called mean absolute error in the measurement of that physical quantity.
∆amean = Δa1+Δa2+…….+Δann=1n∑ni=1Δai
Relative error:
The ratio of the mean absolute error in the measurement of a physical quantity to its arithmetic mean value is called relative error.
Relative error = Δameanamean
Percentage error:
The relative error represented by percentage (i.e., multiplied by 100) is called the percentage error.
Percentage error = Δameanamean × 100%
[Note: Considering conceptual conventions question is modified to define percentage error and not mean percentage error.]
Question 4.
Describe what is meant by significant figures and order of magnitude.
Answer:
Significant figures:
Significant figures in the measured value of a physical quantity is the sum of reliable digits and the first uncertain digit.
OR
The number of digits in a measurement about which we are certain, plus one additional digit, the first one about which we are not certain is known as significant figures or significant digits.
Larger the number of significant figures obtained in a measurement, greater is the accuracy of the measurement. The reverse is also true.
If one uses the instrument of smaller least count, the number of significant digits increases.
Rules for determining significant figures:
All the non-zero digits are significant, for example if the volume of an object is 178.43 cm3, there are five significant digits which are 1,7,8,4 and 3.
All the zeros between two nonzero digits are significant, eg., m = 165.02 g has 5 significant digits.
If the number is less than 1, the zero/zeroes on the right of the decimal point and to the left of the first nonzero digit are not significant e.g. in 0.001405, significant. Thus the above number has four significant digits.
The zeroes on the right hand side of the last nonzero number are significant (but for this, the number must be written with a decimal point), e.g. 1.500 or 0.01500 both have 4 significant figures each.
On the contrary, if a measurement yields length L given as L = 125 m = 12500 cm = 125000 mm, it has only three significant digits.
Order of magnitude:
The magnitude of any physical quantity can be expressed as A × 10n where ‘A’ is a number such that 0.5 ≤ A < 5 then, ‘n’ is an integer called the order of magnitude.
Examples:
Speed of light in air = 3 × 108 m/s
∴ order of magnitude = 8
Mass of an electron = 9.1 × 10-31 kg
= 0.91 × 1030 kg
∴ order of magnitude = -30
Maharashtra Board Class 11 Physics Solutions Chapter 1 Units and Measurements
Question 5.
If the measured values of two quantities are A ± ∆A and B ± ∆B, ∆A and ∆B being the mean absolute errors. What is the maximum possible error in A ± B? Show that if Z = AB
ΔZZ=ΔAA+ΔBB
Answer:
Maximum possible error in (A ± B) is (∆A + ∆B).
Errors in divisions:
i) A
Suppose, Z = AB and measured values of A and B are (A ± ∆A) and (B ± ∆B) then,
Maharashtra Board Class 11 Physics Solutions Chapter 1 Units and Measurements 5
Maharashtra Board Class 11 Physics Solutions Chapter 1 Units and Measurements 6
∴ Maximum relative error of ΔZZ=±(ΔAA+ΔBB)
ii) Thus, when two quantities are divided, the maximum relative error in the result is the sum of relative errors in each quantity.
Question 6.
Derive the formula for kinetic energy of a particle having mass m and velocity v using dimensional analysis
Answer:
Kinetic energy of a body depends upon mass (m) and velocity (v) of the body.
Let K.E. ∝ mx vy
∴ K.E. = kmx vy …….. (1)
where,
k = dimensionless constant of proportionality. Taking dimensions on both sides of equation (1),
[L2M1T-2] – [L0M1T0]x [L1M0T-1]y
= [L0MxT0] [LyM0T-y]
= [L0+yMx+0T0-y]
[L2M1T2] = [LyMxT-y] …………. (2)
Equating dimensions of L, M, T on both sides of equation (2),
x = 1 and y = 2 ,
Substituting x, y in equation (1), we have
K.E. = kmv2
3. Solve numerical examples.
Question 1.
The masses of two bodies are measured to be 15.7 ± 0.2 kg and 27.3 ± 0.3 kg. What is the total mass of the two and the error in it?
Answer:
Given: A ± ∆A = 15.7 ± 0.2kg and
B ± ∆B = 27.3 ± 0.3 kg.
To find: Total mass (Z), and total error (∆Z)
Formulae: i. Z = A + B
ii) ±∆Z = ±∆A ± ∆B
Calculation: From formula (i),
Z = 15.7 + 27.3 = 43 kg
From formula (ii),
± ∆Z (± 0.2) + (± 0.3)
=±(0.2 + 0.3)
= ± 0.5 kg
Total mass is 43 kg and total error is ± 0.5 kg.
Question 2.
The distance travelled by an object in time (100 ± 1) s is (5.2 ± 0.1) m. What is the speed and it’s relative error?
Answer:
Given: Distance (D ± ∆D) = (5.2 ± 0.1) m,
time(t ± ∆t) = (100 ± 1)s.
To find: Speed (v), maximum relative error (Δvv)
Formulae: i. v = Dt
ii. Δvv=±(ΔDD+Δtt)
Calculation: From formula (i),
v = 5.2100 = 0.052 m/s
From formula (ii),
Δvv=±(0.15.2+1100)
= ±(152+1100)=±19650
= ± 0.029 rn/s
The speed is 0.052 m/s and its maximum relative error is ± 0.029 m/s.
[Note: Framing of numerical is modified to make it specific and meaningful.]
Maharashtra Board Class 11 Physics Solutions Chapter 1 Units and Measurements
Question 3.
An electron with charge e enters a uniform. magnetic field B⃗ with a velocity v⃗ . The velocity is perpendicular to the magnetic field. The force on the charge e is given by
|F⃗ | = Bev Obtain the dimensions of B⃗ .
Answer:
Given: |F⃗ | = B e v
Considering only magnitude, given equation is simplified to,
F = B e v
Maharashtra Board Class 11 Physics Solutions Chapter 1 Units and Measurements 3
∴ B = [L0M1T-2I-1]
[Note: The answer given above is calculated in accordance with textual method considering the given data.]
Question 4.
A large ball 2 m in radius is made up of a rope of square cross section with edge length 4 mm. Neglecting the air gaps in the ball, what is the total length of the rope to the nearest order of magnitude?
Answer:
Volume of ball = Volume enclosed by rope.
43 π (radius)3 = Area of cross-section of rope × length of rope.
∴ length of rope l = 43πr3A
Given:
r = 2 m and
Area = A = 4 × 4 = 16 mm2
= 16 × 10-6 m2
∴ l = 4×3.142×233×16×10−6
= 3.142×23 × 10-6 m
≈ 2 × 106 m.
Total length of rope to the nearest order of magnitude = 106 m = 103 km
Question 5.
Nuclear radius R has a dependence on the mass number (A) as R = 1.3 × 10-16 A13 m. For a nucleus of mass number A=125, obtain the order of magnitude of R expressed in metre.
Answer:
R= 1.3 × 10-16 × A13 m
For A = 125
R= 1.3 × 10-16 × (125)13
= 1.3 × 10-16 × 5
= 6.5 × 10-16
= 0.65 × 10-15 m
∴ Order of magnitude = -15
[Note: Taking the standard value of nuclear radius R = 1.3 × 10-155 m, the order of magnitude comes to be 10-14 m.]
Question 6.
In a workshop a worker measures the length of a steel plate with a Vernier callipers having a least count 0.01 cm. Four such measurements of the length yielded the following values: 3.11 cm, 3.13 cm, 3.14 cm, 3.14 cm. Find the mean length, the mean absolute error and the percentage error in the measured value of the length.
Answer:
Given: a1 = 3.11 cm, a2 = 3.13 cm,
a3 = 3.14 cm. a4 = 3.14cm
Least count L.C. = 0.01 cm.
To find.
i. Mean length (amean)
ii. Mean absolute error (∆amean)
iii. Percentage error.
Formulae: i. amean = a1+a2+a3+a44
ii. ∆an = |amean – an|
iii. ∆amean = Δa1+Δa2+Δa3+Δa44
iv. Percentage error = Δameanamean × 100
Calculation: From formula (i),
amean = 3.11+3.13+3.14+3.144
= 3.13 cm
From formula (ii),
∆a1 = |3.13 – 3.11| = 0.02 cm
∆a2 = |3.13 – 3.13| = 0
∆a3 = |3.13 – 3.14| = 0.01 cm
∆a4 = |3.13 – 3.14| = 0.01 cm
From formula (iii),
∆amean = 0.02+0+0.01+0.014 = 0.01 cm
From formula (iii).
% error = 0.013.13 × 100
= 13.13
= 0.3196
……..(using reciprocal table)
= 0.32%
i. Mean length is 3.13 cm.
ii. Mean absolute error is 0.01 cm.
iii. Percentage error is 0.32 %.
[Note: As per given data of numerical, percentage error calculation upon rounding off yields percentage error as 0.32%]
Maharashtra Board Class 11 Physics Solutions Chapter 1 Units and Measurements
Question 7.
Find the percentage error in kinetic energy of a body having mass 60.0 ± 0.3 g moving with a velocity 25.0 ± 0.1 cm/s.
Answer:
Given: m = 60.0 g, v = 25.0 cm/s.
∆m = 0.3 g, ∆v = 0.1 cm/s
To find: Percentage error in E
Formula: Percentage error in E
(Δmm+2Δvv) × 100%
Calculation: From formula,
Percentage error in E
= (0.360.0+2×0.125.0) × 100%
= 1.3%
The percentage error in energy is 1.3%.
Question 8.
In Ohm’s experiments, the values of the unknown resistances were found to be 6.12 Ω , 6.09 Ω, 6.22 Ω, 6.15 Ω. Calculate the mean absolute error, relative error and percentage error in these measurements.
Answer:
Given: a1 = 6.12 Ω, a2 = 6.09 Ω, a3 = 6.22 Ω, a4 = 6.15 Ω,
To find:
i) Absolute error (∆amean)
ii) Relative error
iii) Percentage error
Formulae:
i) amean = a1+a2+a3+a44
ii) ∆an = |amean – an|
iii) ∆amean = Δa1+Δa2+Δa3+Δa44
iv) Percentage error = Δameanamean × 100
From formula (ii),
∆a1 = |6.145 – 6.12|= 0.025
∆a2 = |6.145 – 6.09| = 0.055
∆a3 = |6.145 – 6.22| = 0.075
∆a4 = |6.l45 – 6.15| = 0.005
From formula (iii),
∆amean = 0.025+0.055+0.075+0.0054=0.1604
= 0.04 Ω
From formula (iv),
Relative error = 0.046.145 = 0.0065 Ω
From formula (v).
Percentage error = 0.0065 \frac{0.04}{6.145} 100 = 0.65%
i. The mean absolute error is 0.04 Ω.
ii. The relative error is 0.0065 Ω.
iii. The percentage error is 0.65%.
[Note: Framing of numerical is modified to reach the answer given to the numerical.]
Question 9.
An object is falling freely under the gravitational force. Its velocity after travelling a distance h is v. If v depends upon gravitational acceleration g and distance, prove with dimensional analysis that v = kgh−−√ where k is a constant.
Answer:
Given = v = kgh−−√
Maharashtra Board Class 11 Physics Solutions Chapter 1 Units and Measurements 4
k being constant is assumed to be dimensionless.
Dimensions of L.H.S. = [v] = [L1T-1]
Dimension of R.H.S. = [latex]\sqrt{g h}[/latex]
= [L1T-2]12 × [L1]12
= [L2T-2]12
= [L1T-1]
As, [L.H.S.] = [R.H.S.],
=> v = kgh−−√is dimensionally correct equation.
Question 10.
v = at + bt+c + v0 is a dimensionally valid equation. Obtain the dimensional formula for a, b and c where v is velocity, t is time and v0 is initial velocity.
Answer:
Solution: Given: y = at + bt+c + + v0
As only dimensionally identical quantities can be added together or subtracted from each other, each term on R.H.S. has dimensions of L.H.S. i.e., dimensions of velocity.
∴ [LH.S.] = [v] = [L1T-1]
This means, [at] = [v] = [L1T-1]
Given, t = time has dimension [T-1]
∴ [a] = [L1 T−1][t]=[L1 T−1][T1] = [L1T-2] = L1M0T-2]
Similarly, [c] = [t] = [T1] = [L0M0T1]
∴ [b][T1] = [v] = [L1T-1]
∴ [b] = [L1T-1] × [T1] = [L1] = [L1M0T0]
Maharashtra Board Class 11 Physics Solutions Chapter 1 Units and Measurements
Question 11.
The length, breadth and thickness of a rectangular sheet of metal are 4.234 m, 1.005 m, and 2.01 cm respectively. Give the area and volume of the sheet to correct significant figures.
Answer:
Given: l = 4.234 m, b = 1.005 m,
t = 2.01 cm = 2.01 × 10-2 m = 0.0201 m
To find:
i) Area of sheet to correct significant figures (A)
ii) Volume of sheet to correct significant figures (V)
Formulae: i. A = 2(lb + bt + tl)
iii) V = l × b × t
Calculation: From formula (i),
A = 2(4.234 × 1.005 + 1.005 × 0.0201 +0.0201 × 4.234)
= 2 |[antilog(log 4.234 + logl .005) + antiiog(log 1.005 + log0.0201) + antilog(log 0.0201 + log 4.234)]}
= 2{[antilog(0.6267 + 0.0021) + antilog(0.0021 + 2¯¯¯ .3010) + antilog (2¯¯¯ .3010 + 0.6267)]}
= 2 {[antilog(0.6288) + antilog (2¯¯¯[/latex.3031)+antilog([latex]2¯¯¯ .9277)]}
= 2 [4.254 + 0.02009 + 0.08467]
= 2 [4.35876]
= 8.71752m2
In correct significant figure,
A = 8.72 m2 From formula (ii),
V =4.234 × 1.005 × 0.0201
= antilog [log (4.234) + log (1.005) + log (0.0201)]
= antilog [0.6269 – 0.0021 – 2¯¯¯.3032]
= antilog [0.6288 – 2¯¯¯.3032]
= antilog [ 2 .9320]
= 8.551 × 10-2
= 0.08551 m3
In correct significant figure (rounding off),
V = 0.086 m3
i.) Area of sheet to correct significant figures is 8.72 m2.
ii) Volume of sheet to correct significant figures is 0.086 m3.
[Note: The given solution is arrived to by considering a rectangular sheet.]
Question 12.
If the length of a cylinder is l = (4.00 ± 0.001) cm, radius r = (0.0250 ± 0.001) cm and mass m = (6.25 ± 0.01) gm. Calculate the percentage error in the determination of density.
Answer:
Given: l = (4.00 ± 0.001) cm,
In order to have same precision, we use, (4.000 ± 0.001),
r = (0.0250 ± 0.00 1) cm,
In order to have same precision, we use, (0.025 ± 0.001)
m = (6.25 ± 0.01) g
To find: percentage error in density
Formulae:
i) Relative error in volume, ΔVV=2Δrr+Δll
….(∵ Volume of cylinder, V = πr2l)
ii) Releative error Δρρ=Δmm+ΔVV
Maharashtra Board Class 11 Physics Solutions Chapter 1 Units and Measurements 10
iii) Percentage error= Relative error × 100%
Calculation.
From formulae (i) and (ii),
∴ Δρρ=Δmm+2Δrr+Δll
= 0.016.25+2(0.001)0.025+0.0014.000
= 0.00 16 + 0.08 + 0.00025
= 0.08 185
From formula (iii).
% error in density = Δρρ × 100
= 0.08185 × 100
= 8.185%
Percentage error in density is 8.185%.
Maharashtra Board Class 11 Physics Solutions Chapter 1 Units and Measurements
Question 13.
When the planet Jupiter is at a distance of 824.7 million kilometers from the Earth, its angular diameter is measured to be 35.72″ of arc. Calculate the diameter of the Jupiter.
Answer:
Given: Angular diameter (a) = 35.72″
= 35.72″ × 4.847 × 10-6 rad
1.73 × 10-4 rad
Distance from Earth (D)
= 824.7 million km
= 824.7 × 106 km
Calculation: From formula,
d = 1.73 × 10-4 × 824.7 × 109
= 1.428 × 108 m
= 1.428 × 105 km
Diameter of Jupiter is 1.428 × 105 km.
Question 14.
If the formula for a physical quantity is X =
Answer:
Given X =
Percentage error in a, b, c, d is respectively 2%, 3%, 3% and 4%.
Now, Percentage error in X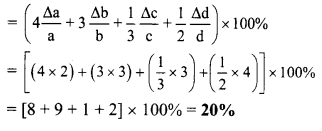
Question 15.
Write down the number of significant figures in the following: 0.003 m2, 0.1250 gm cm-2, 6.4 × 106 m, 1.6 × 10-19 C, 9.1 × 10-31 kg.
Answer:
Question 16.
The diameter of a sphere is 2.14 cm. Calculate the volume of the sphere to the correct number of significant figures.
Answer:
Volume of sphere =
=
=
= 1.333 × 3.142 × (1.07)3
= {antilog [log (1.333) + log(3.142)+3 log(1.07)]}
= {antilog [0.1249 + 0.4972 + 3 (0.0294)])
= {antilog [0.6221 + 0.0882]}
= {antilog [0.7103]}
= 5.133cm3
In multiplication or division, the final result should retain as many significant figures as there are in the original number with the least significant figures.
Volume in correct significant figures
∴ 5.13 cm3













